I. Introduction

factorial design--two or more independent variables (or factors) are combined into a single study

A notation system is used to convey the number of factors and the number of levels that exist for each factor

 Consider the following example: 

Figure 12.1  The Structure of a Two-Factor Experiment Where Alcohol Consumption (the 1st factor or factor A) and Caffeine Consumption (the 2nd factor or factor B) are Manipulated in the Same Study (p. 293)

How many levels of factor A?  How many levels of factor B?

Therefore, this is a __ x __ design.

Why not do two separate studies, each investigating one of the independent variables? 

    Ask: How does alcohol consumption affect reaction time? or How does caffeine consumption affect reaction time?

Advantages of factorial designs:

(1) less artificial than single-factor designs

(2) enable us to access how IVs interact or combine to influence behavior differently than when considered separately

    Can instead ask: How do changes in caffeine consumption influence the effects of alcohol on behavior? or How do changes in alcohol consumption influence the effects of caffeine on behavior?

II. Main Effects & Interactions

Main effect--the mean differences among the levels of one factor

To assess the main effects for alcohol, which numbers will be compared? Addresses what question?

To assess the main effects for caffeine, which numbers will be compared? Addresses what question?

But is there an alcohol x caffeine interaction? Do the differences between the cells in the matrix (the treatment combination means) show effects that are different from the overall main effects?

Interaction--the unique effects produced by two factors working together

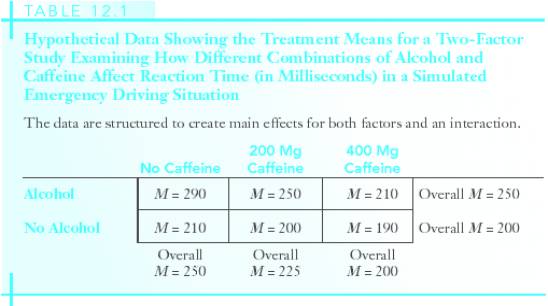
 Table 12.1  Hypothetical Data Showing the Treatment Means for a Two-Factor Study Examining How Different Combinations of Alcohol and Caffeine Affect Reaction Time (in msec) in a Simulated Emergency Driving Situation (p. 296)

**How do we describe this interaction in words?

 Table 12.2  Hypothetical Data Showing the Treatment Means for a Two-Factor Study Examining How Different Combinations of Alcohol and Caffeine Affect Reaction Time (in msec) in a Simulated Emergency Driving Situation (p. 298) 

 Figure 12.3  A Line Graph of the Data from Table 12.2 (p. 301)

 Figure 12.5  Three Possible Combinations of Main Effects and Interactions in a Two-Factor Experiment
(p. 304)